 |


Domitius Ulpianus was a celebrated Roman jurist who lived during the same period as Dio Cassius (c. 150–235). His ancestors came from Tyre, Phoenicia. Some of his works on law may have been written in the joint reign of Septimus Severus and his son Antonius Caracalla (AD 211) during which time Ulpian was Prefect of the Praetorian Guard, that is, commander of the emperor's guard. The greater part of his legal writings was written during the sole reign of Caracalla (212-217). Under Elagabalus (218-222), Ulpian was banished. He returned to Rome in 222 to become chief advisor to Emperor Alexander Severus. Ulpian was killed by his enemies in the Praetorian Guard in 228.
Under Justinian, emperor at Constantinople from 527 to 565, the existing Roman law was consolidated. The result, known as the Corpus Juris of Justinian, consists of four parts: the Institutes (Institutiones), the Digest (Digesta), the Code (Codex) and the Novels or New Laws (Novellae). The Institutes, published in 533, is a short manual or textbook on the whole law. The Digest, also published in 533, consists of extracts from authoritative jurists organized by subject. The Code collects the laws of the emperors and their responses to legal questions. It was first published in 529 but a revised edition appeared in 534. Finally, the Novels contain the laws enacted under Justinian himself. A large collection of material related to Roman Law is accessible at The Roman Law Library. The Liberty Library hosts Scott's somewhat out-dated but still useful Civil Law published in 1932.
Ulpian's writings survive only as extracts within the Digest. The judgments of Ulpian were so definitive that the number of excerpts from his writings found there is said to be 2462, or about one-third of the total.
The particular extract of interest by Ulpian is that quoted by Aemilius Macer, another Roman jurist who worked during the reign of Alexander Severus (222-235). Macer wrote several works from which extracts are given in the Digest of Justinian. One in particular is Ad Legem de Vicesima Hereditatum, On the Law of the Inheritance Tax or, literally, On the Law of the Twentieth Portion of an Inheritance. It is from this work that the following is drawn, its purpose being to give a modification of the rule used in the determination of the value of a usufruct. Of special importance was its use in the computation of the so-called Falcidian Fourth, the minimal share of an estate awarded to an heir. Niklaus Bernoulli discusses this topic and its relationship to Ulpian's rule at length in Chapter V of his doctoral dissertation, the De Usu Artis Conjectandi in Iurus of 1709.
This same extract is of interest for another reason. Some have claimed that it represents an early table of life expectancy.
CORPUS IURIS CIVILIS. I. DIGESTA. XXXV. 2.68
Aemilius Macer, On the Statute of the Five Percent Inheritance Tax, book 2.
Ulpian writes that this is the formula for a computation to be made concerning maintenance, that:
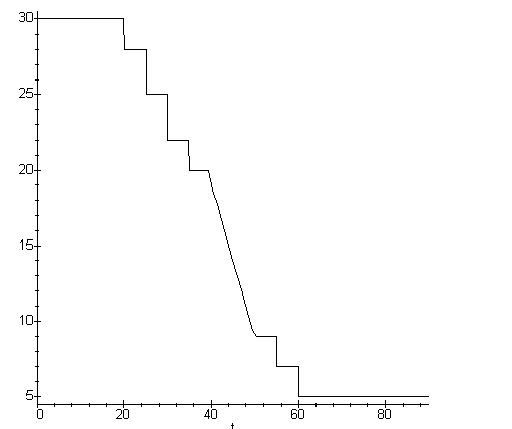
from birth to the twentieth year the amount of maintenance is computed as thirty years, and the Falcidian <fourth> of this amount is answered for;
from twenty years indeed up to the twenty-fifth, twenty-eight years;
from twenty-five years up to thirty years, twenty-five years;
from thirty years up to thirty-five years, twenty-two years;
from thirty-five years up to forty years, twenty years;
from forty years up to fifty years, a computation is made for so many years, as many as are wanting in his age compared with the sixtieth year, with one year subtracted;
from the fiftieth year indeed up to the fifty-fifth year, nine years;
from fifty-five years up to the sixtieth year, seven years;
from sixty years to whatever age he might be, five years.
Ulpian states that we make use of this law also in respect to the computation of a usufruct. Nevertheless it is customary from birth up to the thirtieth year, that a computation of thirty years be made: from thirty years indeed, so many years, as many as seem to be wanting compared to the sixtieth year: therefore a computation never more than thirty years is entered upon. So equally in the case of a legacy of a usufruct to the state, whether simply for the provision of games or without restriction, the valuation will be of thirty years.
It is easy to extract from this passage the life expectancy for one of any given age and to plot this same function. A serious flaw with respect to its interpretation as a life function is that it assigns five more years of life to all over age 60. This is unrealistic.
| Age | [0,20) | [20,25) | [25,30) | [30,35) | [35,40) | [40,50) | [50,55) | [55,60) | 60+ |
| Remaining Life |
30 |
28 |
25 |
22 |
20 |
59-Age |
9 |
7 |
5 |
 |
It is possible to construct the distribution of the number who remain living at each age of a cohort of births from the life expectancy function. If, for example, there is assumed 1000 births then the distribution of the number yet living at each age appears as shown in the figure below. It is should be clear that this distribution can belong to no real population because it is not monotone decreasing.
|
|
One should note that the attribution to Ulpian of a life table is but pure speculation. There is no evidence that Ulpian had anything as sophisticated as this in mind.
For interesting discussions see: