Commentary
on
De Usu Artis Conjectandi in Iure

Richard J. Pulskamp

October 10, 2009

1  Introduction

Historians of probability have called attention to the 1709 dissertation De Usu Artis Conjectandi in Iure of Niklaus Bernoulli. Yet no translation of this work into any modern language has been published. The present intent is to remedy this situation.

Niklaus I Bernoulli was born in Basel, Switzerland 21 October 1687. His father, Niklaus Bernoulli, was the brother of Jakob I (1654–1705) and Johann I (1667–1748). Niklaus I attended the University of Basel where he studied mathematics under his uncles. He earned his master’s degree in 1704 for his defence of Jakob’s last paper on infinite series, "De seriebus infinitis earumque usu in quadraturis spatiorum et rectificationibus curvarum." The dissertation of 1709 earned him the doctor of jurisprudence.

In 1716 Niklaus I became professor of mathematics at Padua, but he returned to the University of Basel in 1722 to accept the chair of logic. This he exchanged for a professorship in law in 1731. He remained there until his death 29 November 1759.

In order to place the dissertation in context, it is necessary to first turn attention to his two uncles. Jakob was formally trained in philosophy and theology but studied mathematics in spite of efforts by his father to the contrary. In 1677 he began writing his scientific diary, the Meditationes, while serving as a tutor in Geneva. Jakob returned Basel in 1683 where he lectured as magister artium (master of arts) on experimental physics. He continued to study recent mathematical works and published papers on science and mathematics in both the Journal des sçavans and the Acta eruditorum. In 1687 he became professor of mathematics and held this position until his death.

In 1683 Johann came to the University of Basel where he studied mathematics privately under Jakob. He became magister artium in 1685 and then began the study of medicine. His dissertation De motu musculorum of 1694 is actually a mathematical work. Jakob and Johann were the first to truly master the infinitesimal calculus of Leibniz. When Jakob died in 1705, Johann immediately succeeded to his chair in mathematics.

Jakob’s contribution to probability is the Ars Conjectandi. It consists of four parts, the last remaining unfinished. Part I reprints the Tractatus de ratiociniis in aleae ludo of Christiaan Huygens together with a valuable commentary. The de ratio. was first published in 1657 as an appendix to the Exercitationes mathematicae of Franz van Schooten. The second part concerns the theory of permutations and combinations. The third gives applications of the previous to games of chance. The unfinished last portion is the most original.

The purpose of Part IV was to demonstrate the use of the art of conjecture in civil and moral affairs. It contains philosophical thoughts on probability, a definition of moral certainty and a proof of what is now called Bernoulli’s theorem.

At what point Jakob began writing the Ars Conjectandi is not clear. Jakob’s diary of 1684–1685 contains his solutions to each of the five problems posed by Huygens. Until 1689 entries are found in which he considers permutations, combinations, and problems related to chance. Certainly he had proved Bernoulli’s theorem no later than 1692. In a letter to Leibniz 20 April 1704 he states that he had shown the demonstration to Johann twelve years earlier.

Jakob never found a way to complete Part IV. Niklaus claims it was due to ill health and ultimately his death. Because Niklaus was quite familiar with the art of conjecture, he was asked to complete it. He declined by reason of his youth and inexperience Nonetheless he recommended that the treatise be published as it stood and it was finally printed in 1713.

The purpose of the De Usu is to show how the art of conjecture can be applied to civil and moral affairs. Niklaus shows how to compute life expectancy for individuals and joint lives, how to properly evaluate annuities, alimony, usufructs and assurance. He examines lotteries and shows how to compute naval interest. Of more speculative nature concerns his thoughts on declaring a missing one for dead, the number of offspring in a pregnancy, the credibility of witnesses and commodation.

In reading the De Usu it is quite clear how indebted Niklaus is to Jakob. Not only has he drawn from the Ars Conjectandi both literally and for inspiration, but he has taken from the Meditationes and a work on logic, Dissertatione de Conversione & Oppositione. Niklaus does not always credit his sources. Sometimes direct quotations are indicated, in others the reference is oblique. Still many verbatim extracts are not indicated as such at all.

The text of the De Usu has been published in the collected works of Jakob Bernoulli: Vol. 3 Wahrscheinlichkeitsrechnung (1975). In preparing the translation of the De Usu it was decided to retain to the greatest extent as is reasonable the formatting of the text as published there. However, the dissertation runs continuously except for chapter breaks. For the most part direct quotations and references are italicized. For the convenience of the reader, paragraphs have been created at natural points and the quotations have been set off from the text. The numbers in the margin approximate as closely as possible the original pagination. Footnotes have been introduced to give the sources of the extracts and to explicate obscure points in the text.

Niklaus studied Roman law. The references to the Corpus Iuris Civilis and to the other legal literature have not been translated. Other references have however. A list of authorities give the full name, dates, and a brief comment of each source as published with the collected works of Jakob.

2  Notes

All translations from the Ars Conjectandi are by Bing Sung. The section numbers preceding paragraphs refer to the original pagination as noted in the margins of the De Usu or correspondingly to the cited works.

2.1  Chapter I: The art of conjecture in general

§5. In the work of Huygens, the concern is expectation or odds, not probability. In this selection from the Ars Conjectandi we have certainty denoted by 1 so that probability can now be represented as a pure number.

The Rule for probability beginning “Multiplicetur id quod…" is not a direct quote. It can be considered a paraphrase of Proposition III of Huygens.

What is quite interesting here is the analogy to the Rule of Mixtures, computation of center of gravity and the mean. 

2.2  Chapter II: Life Expectancy

Niklaus makes use of extracts from Article 77 of the Mediationes and the Ars Conjectandi without credit. 

§9. The mortality table taken from the Dissertatione de Conversione & Oppositione is that of John Graunt. Graunt presented to the Royal Society in January of 1662 his Natural and Political Observations on the Bills of Mortality. Graunt’s table is not a true mortality table. Graunt estimated the number of deaths within the first 6 years by seeking out those deaths given in the bills of mortality due to the diseases of childhood. He writes in Chapter XI:

§9. Where as we have found, that of 100 quick Conceptions about 36 of them die before they be six years old, and that perhaps but one surviveth 76, we, having seven Decads between six and 76, we sought six mean proportional numbers between 64, the remainer, living at six years, and the one, which survives 76, and finde, that the numbers following are practically near enough to the truth; for men do not die in exact Proportions, nor in Fractions: from when arises this Table following.
Viz. of 100 there dies within the first six years  36
The next ten years, or Decad 24
The second Decad 15
The third Decad 9
The fourth 6
The next 4
The next 3
The next 2
The next 1

§10. From whence it follows, that of the said 100 conceived there remains alive at six years end 64.

At Sixteen years end  40
At Twenty six 25
At Thirty six  16
At Fourty six 10
At Fifty six  6
At Sixty six 3
At Seventy six  1
At Eighty  0

§11. It follows also, that of all, which have been conceived, there are now alive 40 per Cent. above sixteen years old, 25 above twenty six years old, & sic deinceps, as in the above Table: there are therefore of Aged between 16, and 56, the number of 40, less by six, viz. 34; of between 26, and 66, the number of 25 less by three, viz. 22: & sic deinceps.

Robert Moray sent a copy of the Observations to Christiaan Huygens on 16 March 1662. Nothing came of this until Christiaan and his brother Ludwig exchanged correspondence concerning the use of the table to compute life expectancy. In Ludwig’s letter dated 30 October 1669 (No. 1771), he shows how he computes the life expectancy of a newborn, 6 year old, …, 76 year old. He further remarks that for someone between these ages linear interpolation is used to compute life expectancy.

Christiaan replies in a letter of 21 November 1669 (No. 1776). He notes that for the purpose of wagering about life expectancy one should use percentiles rather than means. In particular, while a newborn infant may have an expected lifetime of 18.22 years there are even odds the child will survive to 11 years of age.

In his Adversaria or notebook, Christiaan completes this own computations of life expectancy which are seen to agree with Ludwig’s. Of interest are several additonal problems:

  1. In order to know in what time 2 die of 40 persons age 46 years. Answer. 1 year 3 months.
  2. A man of 56 years marries a woman of 16 years. How long are they able to make a life together without one or the other dying? Or else, if one promised me 100 francs at the end of each year they will live together, for how much will it be exactly to buy back this obligation? Likewise, in how many years will the two die?
  3. In how many years will 40 men die of 46 years each?
  4. In how many years will die 2 persons of age 16 years each. Answer 29 years, 22/3 months.

These questions are communicated to Ludwig in a letter dated 28 November 1669 (No. 1781)

In the Philosophical Transactions of the Royal Society of 1693 is found the paper of Edmund Halley entitled the “Degrees of mortality of mankind." This contains the famous mortality table derived from the records of Breslau (Wroclaw) Poland.

Under the assumption that the population of Breslau had been a stationary population for many decades, Halley concluded that a table which displayed the number of people at each age must also display the chances of mortality at each age. An analysis of the Breslau data permitted Halley to construct the following table. This table shows the number of individuals living at each age.

Age No. Age No. Age No. Age No. Age No. Age No.
1 1000 8 680 15 628 22 586 29 539 36 481
2 855 9 670 16 622 23 579 30 531 37 472
3 798 10 661 17 616 24 573 31 523 38 463
4 760 11 653 18 610 25 567 32 515 39 454
5 732 12 646 19 604 26 560 33 507 40 445
6 710 13 640 20 598 27 553 34 499 41 436
7 692 14 634 21 592 28 546 35 490 42 427
Age No. Age No. Age No. Age No. Age No. Age No.
43 417 50 346 57 272 64 202 71 131 78 58
44 407 51 335 58 262 65 192 72 120 79 49
45 397 52 324 59 252 66 182 73 109 80 41
46 387 53 313 60 242 67 172 74 98 81 34
47 377 54 302 61 232 68 162 75 88 82 28
48 367 55 292 62 222 69 152 76 78 83 23
49 357 56 282 63 212 70 142 77 68 84 20

Niklaus appears to have been unaware of both Huygens’ and Halley’s work.

In the Ars Conjectandi Part IV Chapter II Axiom 4 we have

Remote and general proofs are sufficient for judging about general events; but for forming conjectures about specific events, more closely related and special proofs must be added, if only they can be obtained. And so, when it is asked abstractly how much more probable it is that a young man of twenty years will outlive an old man of sixty years than that the latter will outlive the former, there is nothing besides the difference of age and years which you can consider; but when the conversation is particularly about the specific young man Peter and the specific old man Paul, one must attend moreover to their individual complexions and the concern with which each looks after his own health. For if Peter is sickly, if he indulges in his fancies, if he lives intemperately, it can happen that Paul, although more advanced in age, may nevertheless most reasonably be able to foster hope for a longer life.

§10. Let lx denote the number living at time x and let ex denote the expected future lifetime at age x. Niklaus shows how to compute ex through the equation 

ex={(lx−lx+1)/2+lx+1(1+ex+1)}/lx

For Graunt’s table we want the modified form

ex={5(lx−lx+10)+lx+10(10+ex+10)}/lx

with e76=5 which is sufficiently accurate for our purposes.

§12. The two adages appear in Article 77 of the Meditationes. Here Jakob discusses computation of the mediam of barometric observations. The mediam is what we today would call the arithmetic mean. He says the mediam is what the Germans call eine in die andere gerechnet, the French l’une portant l’autre.

As mentioned above, Christiaan Huygens takes great pains to distinguish between mean life expectancy (which he says is useful for annuities) and median life expectancy (useful for wagers).

§13. Here Niklaus determines the expected value of a maximum. This result is quite striking and completely new.

§16. Unfortunately Bernoulli does not give the original mortality table obtained from his friend. The table can be reconstructed from the first equation if rewritten as

lx+1=lx·(2ex−1)/(2ex+1+1)

Since the interval between successive expected future lifetimes is 5 years, we modifiy the third equation to obtain the reasonable approximation

lx+5=lx·(2ex−5)/(2ex+5+5)

Taking l0=2000, using the fourth equation we have

x 0 5 10 15 20 25 30 35 40 45 50 55 60 65 70 75 80 85
ex 27 38 37 33 30 27 25 22 20 18 15 12 10 8 7 5 4 3
lx 2000 1210 1087 1057 992 924 824 756 656 560 496 427 325 232 134 81 31 8

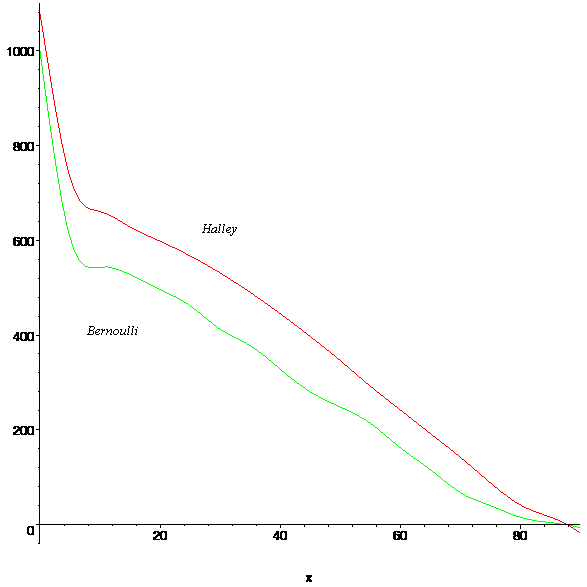
A comparison of this table to Halley’s indicates close agreement. Indeed, in the figure below, the plot of number living by Halley (red) parallels the number living of Niklas (green).

2.3  Chapter III: The absent person considered as dead

§17. In the Ars Conjectandi Part IV Chapter II Axiom 3 we have

One must attend not only to those things which serve to prove the thing, but also to all those things which can be adduced to prove the opposite of the thing, so that after both sides are weighed it will be clear which of them has more weight. It is asked about a friend who has been away from his homeland for a very long time whether he can be declared dead. These proofs serve for the affirmative: that although every effort has been made, nothing has become known of him for all of twenty years; that men who wander about are exposed to a great many perils of life, perils from which men who remain at home are exempt; that therefore he may have ended his life in the sea, he may have died on the road, he may have been killed in battle, he may have perished from disease or misfortune in some place where he was known by no one; that if he were among the living, he must now be of that age which few men reach even if they have lived at home; that he would have written even if he dwelled along the outermost regions of India because he knew that an inheritance awaited him at home; and there are other proofs, but rather the following proofs, which support the negative, must be set forth in opposition: It is well-known that the man was lazy, took hold of the pen painfully, and held his friends in contempt; perhaps he was led away as a captive of the Barbarians so that he was not able to write; perhaps also he wrote several times from India, but the letters were lost due either to the carelessness of the letter-carriers or to the shipwreck of the mailboat; finally, it is well-known that many people have remained away for a longer time and nevertheless have finally returned safe and sound.

Similarly in Article 77b of the Meditationes, item 10.

10. In twenty years time nothing is known from a travelling loved one: it is asked which be more probable, the loved one to be living or to have died? Response: Everyone is judged by conjecture.
These argue for death:
  1. If he was alive certainly he would indicate by letters, even if he lives on an extreme shore of India. 
  2. How often he encounters death by some crisis during the wandering? by chance he ends life in a wave, by chance murder on a road, by chance in battle, by chance in bad luck or by some chance it is met in a place where he is known to nobody.
For life: It is clear that to be inactive, to have seized a pen reluctantly, to scorn friends, by chance being lead into captivity by barbarians; by chance he writes from India, twice or even three times, but the letters are lost either by neglect of being carried away, or by shipwreck. (it is clear many men to stay away a longtime, & yet finally to have returned unexpectedly). [Here Jakob has a note in the margin: he himself knew the inheritance to expect from the household, whereby he wrote vid. Disp. Jurid. Dni. Buntz De Absente pro mortuo declarando.]

NB In civics and in customs actually, not only is the number of cases not determined but it is not known, nor by how much more easily or more difficult one is able to happen over the other, as here: (and in those problems it is ™pšcein (held) entirely to decide which on behalf of one of two; if nevertheless reason itself sent plus de penchant (feels greater tendency) by weighing all circumstances of time, place, of characters, to establish one of the two more probable, the more moderate ought to happen, whereby nothing irrelevant to a critic (only the more bold of mathematicians, & more confident, the more moderate of the remainder) who for the explanation of some word, account, contend as it were before altars & hearths: when conjectures would be caused to be weighed on a balance. or some number of cases had been determined, but he is ignorant of how much would be the probability.
See also the article Absent in the Encyclopédie Méthodique.

2.4  Chapter IV: Annuities

The price of an annuity was usually determined as a fixed fraction of the price of a redeemable annuity or perpetuity. The price of a redeemable could be computed easily from the current interest rate. Typically, an annuity on one life was 2/3 the price of a perpetuity. No consideration of age or health was made for the annuitant; everyone paid the same price. The price was expressed as a multiple of income of 1 unit per year. In Amsterdam redeemables were bought at 25 years purchase based upon an interest rate of 4%. There life annuities were sold at 14 years purchase.

The Dutch and English work on annuities appears to have been unknown to Niklaus.

Jan Hudde (1628–1704) was aware of the correspondence of Christiaan Huygens. He also knew Graunt’s table was useless and drew up his own table of mortality based upon annuitants in Amsterdam from 1586–1590. He and Jan de Witt (1625–1672) used this mortality table to show that the price of life annuities should be set much higher than 14 years purchase. The treatise of Jan de Witt, Waardye van Lyf-renten naer proportie van Losrenten, published in 1671, was the first to base the price of life annuities on any life table.

In his paper, Halley too discusses the valuation of annuities on one life, two lives and so forth. He computes the cost of annuities on one life at 6% per annum giving the table.

Age Years Purchase Age Years Purchase Age Years Purchase
1 10.28 25 12.27 50 9.21
5 13.40 30 11.72 55 8.51
10 13.44 35 11.12 60 7.60
15 13.33 40 10.57 65 6.54
20 12.78 45 9.91 70 5.32

§35. Each class forms a tontine.

2.5  Chapter V: The Falcidian fourth

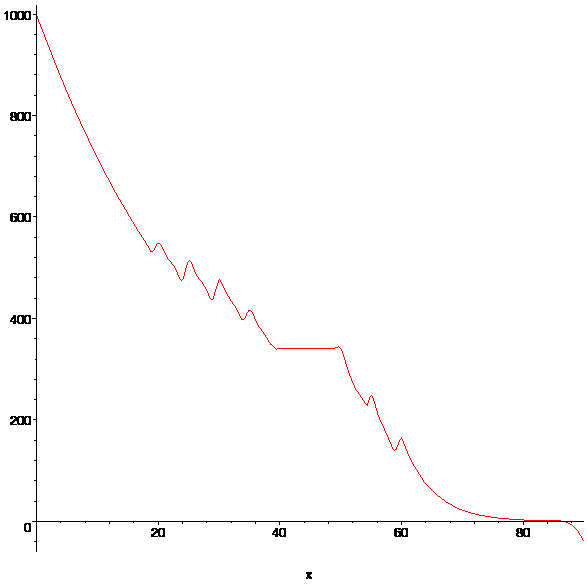
Ulpian’s table is a table of expected future lifetime. Again using the fourth equation, one can reconstruct a life table from the expectation. What is apparent is that Ulpian’s table cannot be derived from any life table for the simple reason that the number of living lx is not monotone decreasing. Taking l0=1000, we have

2.6  Chapter VI: Assurance

§44. From the Ars Conjectandi Part IV Chapter II Axiom 2 we have

2. It is not enough to weigh one or another proof, but everything must be sought out which can come within our realm of knowledge and which appears to have any connection at all with proving the thing. For example: three ships set sail from port; after some time it is announced that one of them suffered shipwreck; which one is guessed to be the one that was destroyed? If I considered merely the number of ships, I would conclude that the misfortune could have happened to each of them with equal chance; but because I remember that one of them had been eaten away by rot and old age more than the others, had been badly equipped with masts and sails, and had been commanded by a new and inexperienced captain, I consider that this ship, more probably than the others, was the one to perish.

Jakob gives a slightly different version of the ship problem in the Meditationes Article 77b Item 6. He considers four bundles for which bundles 1, 2, 3 and 4 valued at 1200, 2000, 2400 and 1700 respectively. Jakob does not solve the problem. In Article 87 he first shows how to compute C(100,20), that is 100 choose 20, and then considers briefly how many of the four bundles may be salvaged among the twenty.

§45. Jakob brings up the problem of nautical interest in Article 77b Item 11 (1685/86) of the Meditationes.

How much would be the just price paid that will be undertaken of some danger of shipwreck; which concerns nautical interest, see Dissert. Dni. Edzards de Naufragio.

2.7  Chapter VII: Lotteries

The Genoise lottery held a certain fascination. Even Euler wrote a paper on it. Jakob discusses the lottery in Article 89 (1688) of the Meditationes.

In Genoa when the senators are elected by chance from a certain number of nobles, they are who are uncovered in a certain kind of Glückhafen, this is the reason: they commend a list of all candidates on the wall, and from this source thence examples also are released to scattered cities, to anyone already pleasurably it is his fortune to try, he throws together those three from such number on the list, which he casts by writing his own name to the author of the Glückhafen, with a moderate monetary payment; and again from him he receives in exchange an emblem (a token). Now if presently in the election of the senators fate will bring, as one of these three being chosen, there is paid to him a certain sum of money, from which it had been agreed; if fortune favors two, more; if all three, yet more; if none of these, the price paid makes a loss. Question: how great a price and to each case ought to be established, as would strive for equal lot?

Let the number of all candidates=a=100.

The smaller number of elected=b=8.

The names of the the three noted in the schedule A,B,C.

The money released to the author=h=12.

The established payment, if one in the list is elected =x
if two =y
if three =z

The number of lots, of which they are able to elect from a−3…

Now put for the sake of brevity the number of cases

of which two in the list are elected=d=(a−b)× 3c/b−2

of which one is elected=e=(a−b−1)×(a−b)× 3c/(b−1)×(b−2)

& of which none=f=(a−b−2)×(a−b−1)×(a−b)× c/b×(b−1)×(b−2)

Therefore there are c cases, by which he acquires z
  d   y
  e   x
& f   0

by which means the expectation of it will be

(c· z+d· y+e· x+f· 0)/(c+d+e+f)=(cz+dy+ex)/(c+d+e+f)

which ought to be equal to h, to the same which he gives up to the author, whence the equality cz+dy+ex=hc+hd+he+hf. Therefore when the problem would be indeterminate, indeed two are able to be supposed at pleasure; however because it is convenient by the nature of the matter, that the prize be proportional reciprocally to the number of cases, i.e. by which reason the cases are fewer, by which the prize would be presented more praiseworthy, thus in place of x· y· z we put one of unknown size z, in the remainder these proportions appearing cz/d & cz/e, whence the equation would be such

[c· z+d·(cz/d)+e·(cz/e)+f· 0]/(c+d+e+f) ,   z=(c+d+e+f)· h/3c

whence

cz/d =y= (c+d+e+f)· h/3d & cz/e =x= (c+d+e+f)· h/3e

Note this again the analogy between this & the rule of mixtures, in which things in given received quantities are mixed to a given price, & the single price is asked.

2.8  Chapter IX: Credibility of Witnesses

Jakob in Article 77 Problem V (1685/86) of the Meditationes.

To measure the degree of trust of someone: Divide the number of cases, in which truth is known to have been spoken, by the sum of the former, and the cases in which falsehood had been observed. Or rather if several men in faithfulness having been approved themselves would present testimony of honesty; others of no less in faithfulness having been approved themselves would accuse of perfidy, divide the number of the former by the sum of both.

(Added at a later date.) N.B. If concerning some problem of art or science they would present testimony, in another way, they hold the matters themselves, in what way if by this fact or account; nevertheless they would examine the weights of the reasons; but yet if I have not the capacity to comprehend the reasons, in the artist in his credence (c+d+e+f)· h/3e art, therefore if at some time an astronomer asserts the earth to have moved, he is more deserving of trust, than 100 inexperienced theologians, who would assert the contrary, if I know, the whole lifetime had been worn away in measuring stars, and in observing phenomena by them. If they would determine from the account, by eye in one witness more trust.

In the Ars Conjectandi Part IV Chapter II Axiom 9, Jakob speaks of the use of moral certainty in law.

Because it is still rarely possible to obtain total certainty, necessity and use desire that what is merely morally certain be regarded as absolutely certain. Hence, it would be useful if, by the authority of the magistracy, limits were set up and fixed concerning moral certainty. I mean, if it were fixed whether 99/100 certainty would suffice for producing moral certainty, or whether 999/1000 certainty would be required. Note that then a judge could not be biased, but he would have a guideline which he would continually observe in passing judgment.
This document was translated from LATEX by HEVEA.